
The perimeter of a quarter circle is a little trickier. Finding the Perimeter of a Quarter Circle
#Circle formula full#
If we want to further break it down into the area of a quadrant, we know that each quadrant is equivalent to:Īnd that’s it! The area of a quarter circle is exactly one-fourth the area of a full circle. If we want to find the area of a semicircle, we would divide that area by two. We know that the equation of the area of the whole circle is A = ℼr². Let's review these two formulas:Īrea = ℼr² Finding the Area of a Quarter Circleįinding the area of a quarter circle starts with finding the area of a whole circle. Solution: Given centre is at origin that is (0,0) and radius is 9 units.Finding the perimeter and area of a quarter circle is a good exercise in working with the two circle formulas you should be aware of: the formulas for the perimeter and area. Solved Question 3: Find the equation of the circle, whose centre is at the origin and radius is equal to 9 units. Solution: Comparing with the standard equation of the circle. Solved Question 2: Find the equation of a circle with, coordinates of the center as (4,2) and radius is equal to 6 cm. The center of the circle is (4, −7) and the radius is 4 cm. Where (a, b) is the center and radius is equal to r.
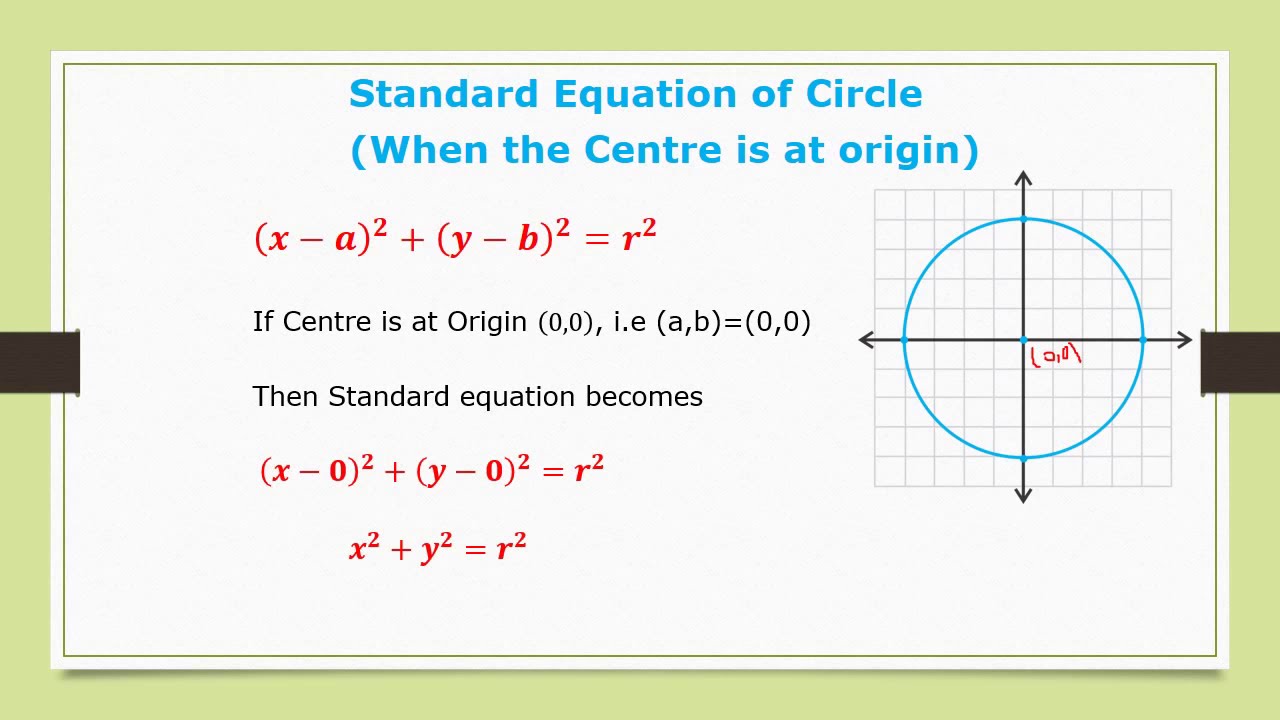
Solved Question 1: If \((x-4)^2+(y+7)^2=16\) is the equation of the circle then, find the center and radius of the circle?Ĭompared with the standard equation of the circle. With the knowledge of circle’s equation through derivation, formulas and various forms let us practice some examples from the same: Solved Examples on Equation of the Circle In polar form, the equation of a circle is always described in the form of r and θ. The polar form of the circle is written as: The parametric equation of a circle is given by the formula: The general equation of any type of circle is represented by: The standard equation of a circle is given by the formula: Then both the coordinates of the center of the given circle are equal to the radius (r, r).Īlso, learn about Vector Algebra here. If in case the circumference of the circle touches both the axes at some point. \((x−r)^2+(y−b)^2=r^2\) Equation of Circle which touches Both the Axes Therefore the coordinates of the center are (r, b). If the given circle touches the y-axis, then the x-coordinate of the center is equal to the radius r. Note that the circle touches the y-axis therefore this is different from where the center of the circle lies on the y-axis. Up next, the situation is when the circle touches the y-axis. Then the distance between this point and the center is given by the formula. Similar to the above cases take (x, y) as an arbitrary point on the circumference of the circle. Therefore the coordinates of the center are (a, r). If the given circle touches the x-axis, then the y-coordinate of the center is equal to the radius r. Note that the circle touches the x-axis therefore this is different from where the center of the circle lies on the x-axis. Next, the situation is when the circle touches the x-axis. Learn the concepts of Three Dimensional Geometry here. If we consider two points (a, b) and (x,y) with r being the radius then:Īpplying the distance formula between these points we get: The equation of a circle can be derived from the distance formula by the following procedure. The standard equation of a circle formula can be derived either by the distance formula or by the Pythagoras theorem as discussed below: Derivation by the Distance Formula Learn about Orthogonal Circles in the linked article. Here (x,y) is an arbitrary point on the circumference of the circle.

The standard equation of a circle with center at (a, b) and radius r. The standard equation of a circle provides accurate information about the center of the circle and its radius making it much easier to understand the center and the radius of the circle at a glance. The line from the centre to one point on the circumference is called the radius. If you form a line, which meets two points on the edge of the circle and goes through the centre, this is named the diameter of the circle and the distance surrounding the circle is termed circumference of the circle (c).

This equation is used over many problems of circles in coordinate geometry. The equation of a circle is given an algebraic approach of defining a circle, given the center and the length of the radius of the circle.


 0 kommentar(er)
0 kommentar(er)
